What to sell

 I have been as Damien says "spread eagle" in trying to cover everything. This can be good or bad depending on what you are trying to do. I have an assignment to do, a presentation to make and an architectural, academic and social audience.

Not everything should be conveyed to all people, this I have touched on in the thought of individualised user catalogues. In this case I am not marketing to the builder or the council but will as most products do advertise to the end user. There are many things the end user will be effected by. I believe what should be presented because of the constraints of the project, is what all markets do and advertise the most popular and most desirable options.

For aneX this means breaking the concept into clear digestible packages for the consumer.

REMEMBER YOU MUST EXHIBIT

"opening of the exhibition will be Friday 11th December 2009 at the Light Square Roma Mitchell Arts Centre

The format of presentation will be:  
One single poster; Width 1682mm x Height 1189mm (NOT NEGOTIABLE)
The poster will need to be finalised and handed into the office no later than 3pm FRIDAY 27th NOVEMBER, in a Jpeg or PDF format, maximum 600dpi, on a CD that we can take to the printers. (you won’t be getting this CD back)

The cost for printing will be $100.00 each person, this is not negotiable and we know that you won’t be able to get your poster printed and hung for a cheaper price.


Everyone will be responsible for hanging their own posters on the Thur 10th December; we have organised to have the assistance of the gallery technician to help arrange posters and lighting." -The Exhibition Committee.

image thanks to - studenttheatre.net.au

Creator Beach House from Lego

Lego Creator(tm) series provides the user with a set of materials and a set of instructions. With this product the User may follow a choice of three instruction sets, to construct one of the three building designs using the set materials.

What is advertised is the big glossy picture of the best looking home you can make. closer inspection reveals the other two possibilities and only once you open the box are the individual components and construction process reveal.


thanks for the image http://www.ohdeedoh.com/ohdeedoh/toys-kids/blogging-cookie-creator-beach-house-from-lego-050239

Module ratios, flexibility experiment #3 - using 5:8:13:21

Here I have continued the exploration in to a finite set of modules. 

The goal is to find a 'smart set' or group of units which 'work well' with each other. I have taken these module units from the Fibonacci sequence as in the previous tests, this time with larger numbers. The qualities I am looking for are the 'flexibility' or ability to form fine graduations in scale.

This combination using 
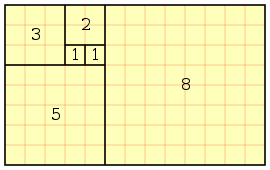
5:8:13:21 appears not to have good flexibility in the initial 10 graduations only 50% of the values can be achieved. However a system that generally uses more than 10 components may not be hindered by this initial small scale short coming. Baring in mind there is no scale set yet so the modules could be meters or millimetres long.

In this ratio there is another short coming I have noticed and left viable in the image.

The value generated by adding 5 and 8 is equal to the next module 13 (because they follow the Fibonacci sequence). The short coming is that the opportunity for a new unit/value from combining two components is wasted as it is the same value as the next size component. this shows an over supply, an excess in the system. This is in itself not a bad thing but when other values are not able to be achieved it is indicating to me that  this ratio is not a 'smart set'.

Ideally the aim is flexibility in the dimensions of an assembled group of components. 

Also there is the fine adjusting which will be a powerful ability of a modular building system, in order to make fine changes to an assembly one would not want to be removing a 21 unit module and replacing it with four 5unit modules. 

The mission for flexibility has failed in many metabolist-esque buildings around the 60's. I believe the problem is in how society is able to control the flexibility, which they must. Defining this flexibility in social terms may be a more appropriate paradigm. The flexibility, physically means the ability to make minute to massive changes with minimal disturbance and do this through time. 

Unique Scheme That Has Huge Potential

text by Prof I.McDougall

Module sizing for minimal unique pieces and maximum flexibility/min graduation

Three different modules;
3, 5, 8 from Fibonacci sequence

The modules used to create graduations of 1 unit from length 3 to 22 except for 7
Images by Will Harbison

"I turned this standalone application into a live example on the web in only 30 minutes"

"Mathematica Solutions for Your Field"

With aneX
image and quote © 2009 Wolfram Research, Inc. 

Phi 1.61803

I am interested in Phi or 'the golden ratio' not for aesthetics but for its functional properties.

Here I am experimenting with the golden ratio and also the Fibonachi sequence (an additive sequence where the next number is the sum of the previous two, ie. 1,1,2,3,5,8...) which approaches the golden ratio.

image thanks to http://en.wikipedia.org/wiki/File:FibonacciBlocks.svg